Pulse Modulation Techniques | PAM, PWM, PCM
Pulse Modulation techniques
Pulse modulation is type of modulation in which the signal is transmitted in the form of pulses. It can be used to transmit analog information. In pulse Modulation, continuous signals are sampled at regular intervals.
Pulse Modulation can be classified into to two major types-
- Analog Modulation
- Digital Modulation
A block diagram showing the basic classification of modulation is given by Fig.
The pulse analog modulation technique are of three types namely, PAM, PWM, and PPM and pulse digital modulation technique are of two types namely DM, DPCM. If amplitude of the pulse is made to the proportional to the message, then it is termed as pulse amplitude modulation(PAM). Alternatively, if the width of the pulse is made proportional to the message, then it is termed s pulse width modulation(PWM). If the position of the pulse train is varied in proportion to the message, then it is termed as pulse position modulation(PPM). Finally, the amplitude of the pulse can be approximately represented by a discrete amplitude value which leads to the pulse code modulation (PCM). Further variants of PCM include delta modulation (DM) and differential PCM (DPCM).
(i) Pulse Analog Modulation
The pulse analog modulation technique are of three types namely, PAM, PWM, and PPM.
(A) Pulse Amplitude Modulation (PAM)
Pulse amplitude modulation is defined as the process of varying the amplitude of the pulse in proportion to the instantaneous variation of message signal. The pulse amplitude modulated signal, will follow the amplitude of the original signal, as the signal traces out the path of the whole wave. In natural PAM, a signal sampled at the Nyquist rate is reconstructed, by passing it through an efficient Low Pass Frequency (LPF) with exact cutoff frequency.
 |
| Waveform representation of PAM signal |
Sampling Process:- Sampling is a signal processing operation that helps in sensing the continuous time signal values at discrete instants of time. The sampled sequence will have amplitudes equal to signal values at the sampling instant and undefined at all other times. This process can be conveniently performed using PAM described above. The continuous time signal to be sampled is applied to the input terminal. The pulse train is applied as the control signal of the switch.
When the pulse occurs, the switch is ON condition that is act as short circuit between input and output terminal. The output value will therefore be equal to input. During the other intervals of the pulse train, the switch is OFF condition that is, act as open circuit. The output is therefore undefined. The output of the switch will be essentially a PAM signal. Any active device like diode, transistor or FET can be used as a switch. The well known sampling theorem which states that the sampling frequency Fs i.e. number of sample per second should be greater than or equal to twice the maximum frequency component Fm of the input signal.
Fs ≥ 2Fm
The minimum possible value of sampling frequency is termed as Nyquist rate. Thus the sampling theorem will decide the periodicity associated with pulse train.
(B) Pulse Width Modulation
Pulse width modulation is defined as a process of varying the width of the pulse in proportion to the instantaneous variation of message. we can see in the figures shown about that the amplitude of the pulse is varying with respect to the amplitude of analogue modulating signal like in case of amplitude modulation. But the major difference is that unlike Amplitude Modulation. Here the carrier is a pulse train rather than continuous wave signal. Therefore, PAM is not useful like AM For communication. Alternatively, PAM is found to be useful in understanding the sampling process to be describe next.
 |
| Waveform representation of PWM signal |
As we can see in the figure shown above that unlike Pulse Amplitude Modulation in this technique the amplitude of the signal is constant and only the width is varying. Pulse width modulation technique is similar to frequency modulation is because, by variation in the width of the pulses the frequency of the pulses in the PWM signal shows variation.
(C) Pulse Position Modulation
Pulse position modulation (PPM) is defined as the process of varying the position of the pulse with respect to the instantaneous variation of the message signal.
 |
| Waveform representation of PPM signal |
Here the pulse amplitude and the Pulse width are the two constant that does not show variation with the amplitude of the modulating signal but only the positions shows variation. It is to be noted here that the positions of the pulse changes according to the reference pulse. And these reference pulses are nothing but PWM pulses.
(ii) Digital Modulation
Pulse digital modulation technique are of two types namely Digital Pulse Code Modulation (DPCM) and Delta Modulation (DM).
(A)Pulse Code Modulation
Pulse code modulationmay be treated as an extension of Pulse Amplitude Modulation. In Pulse Amplitude Modulation the time parameter is discretized, but the amplitude still remains continuous. That is, within the allowable amplitude limits, the signal value can take on infinite values. However all these infinite values may not be distinct from the perception point of view. For instance, in case of signals, all amplitude values may not be important from the auditory perception point of view. Therefore, we may not lost information by descritizing the amplitude to some finite values.
 |
| Block diagram of PCM |
Pulse code modulation is a method that is used to convert an analogue signal into digital signal, so that modified analogue signal can be transmitted through the digital communication network. Pulse Code Modulation is in binary form, so there will be only two possible states high and low (0 and 1). The pulse code modulation process is done in three steps Sampling, quantization and coding. There are two specific types of pulse code modulation such as differential pulse code modulation (DPCM) and adaptive differential pulse code modulation (ADPCM).
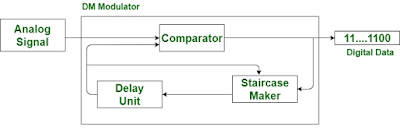
(B) Delta modulation
Delta modulation is obtained by simplifying the quantization and encoding process of PCM. To enable this, the signal is sampled at much higher than the required Nyquist rate. This over sampling process will result the sequence of samples which are very close and hence high co-relations among successive samples. Under this condition it may be safe to assume that may two successive samples are different by an amplitude of δ. That is the current sample is either large or smaller than the previous values by δ. If it is larger, then it is quantized as +δ and -δ delta in smaller case.
The design information can be coded using one bit binary word say 1 present (+) and 0 represent (-) the quantization and and encoding blocks therefore become very simple. If we have the first signal value and 1 bit quantization information we can reconstruct the complete quantization signal.
 |
| Block diagram of DM |


